
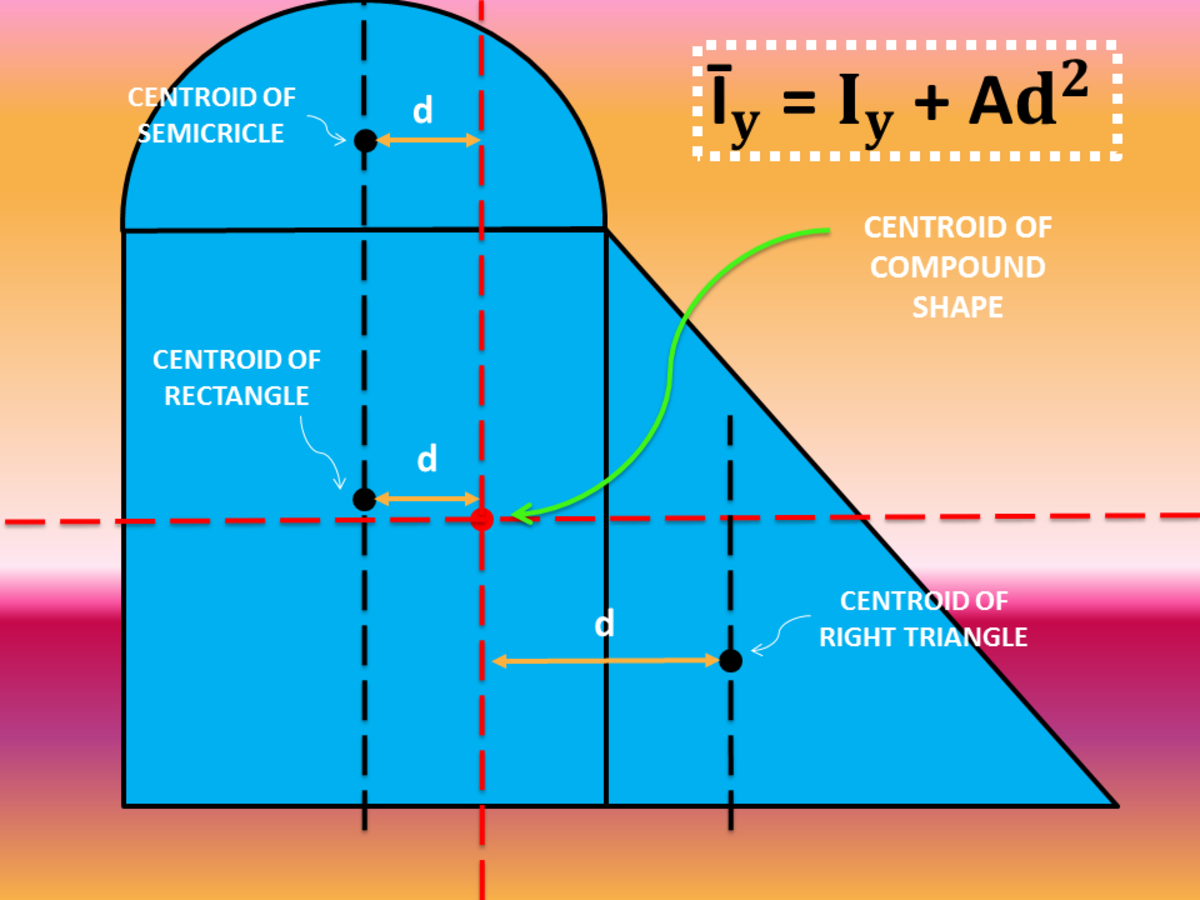
Structural Calculators Properties of Areas Centroid.
The angle that CX’ forms with the x’ axes is φ = 120o - 47.6 o = 72.4 o. The points X’ and Y’ corresponding to the x’ and y’ axes are obtained by rotating CX and CY counterclockwise through an angle Q = 2(60o ) = 120 o.
Based on the circle, evaluate the moments and product of inertia with respect to the x’y’ axes. Construct Mohr’s circle based on the circle diameter between the points. Plot the points (Ix, Ixy ) and (Iy ,-Ixy ). Based on the circle, evaluate the moments and product of inertia with respect to the x’y’ axes. Based on the circle, determine the orientation of the principal axes and the principal moments of inertia. Plot the points (I x, I xy ) and (I y ,-I xy ). 
Using Mohr’s circle, determine (a) the principal axes about O, (b) the values of the principal moments about O, and (c) the values of the moments and product of inertia about the x’ and y’ axes The moments and product of inertia with respect to the x and y axes are Ix = 7.24x106 mm 4, I y = 2.61x106 mm 4, and Ixy = -2.54x106 mm 4. 9.25) and the principal moments of inertia (Eq.
Determine the orientation of the principal axes (Eq. Note that the product of inertia with respect to centroidal axes parallel to the xy axes is zero for each rectangle Compute the product of inertia with respect to the xy axes by dividing the section into three rectangles.Īpply the parallel axis theorem to each rectangle. Compute the product of inertia with respect to the xyaxes by dividing the section into three rectangles and applying the parallel axis theorem to each. Mohr’s circle may be used to graphically or analytically determine the moments and product of inertia for any other rectangular axes including the principal axes and principal moments and products of inertia.įor the section shown, the moments of inertia with respect to the xand yaxes are I x= 10.38 in4and I y= 6.97 in4.ĭetermine (a) the orientation of the principal axes of the section about O,and (b) the values of the principal moments of inertia about O. The moments and product of inertia for an area are plotted as shown and used to construct Mohr’s circle,. 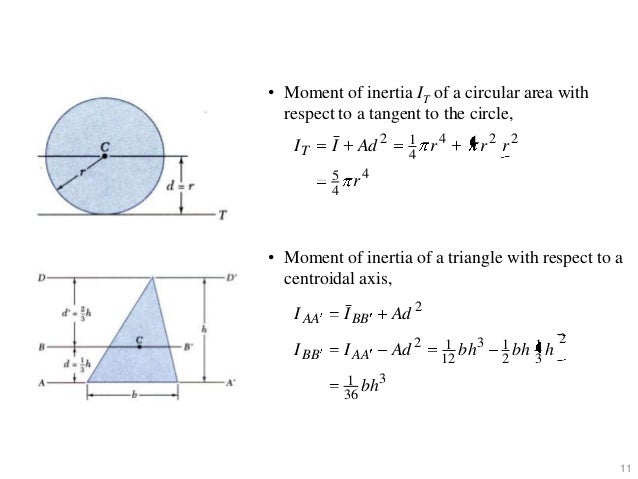
Mohr’s Circle for Moments and Products of Inertia I max and I min are the principal moments of inertia of the area about O.The equation for qm defines two angles, 90o apart which correspond to the principal axes of the area about O.At the points A and B, Ix’y’ = 0 and I x’ is a maximum and minimum, respectively.10-9a and 10-9c and adding, it is found that Principal Axes and Principal Moments of Inertia








 0 kommentar(er)
0 kommentar(er)
